Notes of the book Calculus with Analytic Geometry written by Dr. S. M. Yusuf and Prof. Muhammad Amin, published by Ilmi Kitab Khana, Lahore - PAKISTAN.
Derivative definition The derivative of a function is the ratio of the difference of function value f(x) at points x+Δx and x with Δx, when Δx is infinitesimally small. The derivative is the function slope or slope of the tangent line at point x. By its nature, Calculus can be intimidating. But you can take some of the fear of studying Calculus away by understanding its basic principles, such as derivatives and antiderivatives, integration, and solving compound functions. Also discover a few basic rules applied to Calculus like Cramer’s Rule, and the Constant Multiple Rule, and a few others,. This chapter begins with the definition of the derivative. Two examples were in Chapter 1. When the distance is t2, the velocity is 2t. When f(t) = sin t we found v(t)= cos t. The velocity is now called the derivative off (t). As we move to a more formal definition and new examples, we use new symbols f' and dfldt for the.
Here are few online resource, which are very helpful to find derivative.
Contents and summary
- Geometrical interpretation of the derivative
- Marginal functions in economics
- Inverse trigonometric functions
- Derivative of hyperbolic functions
- Logarithmic Differentiation
- Newtorn Raphson Method
- Some standard nth derivatives
- Functions of several variables
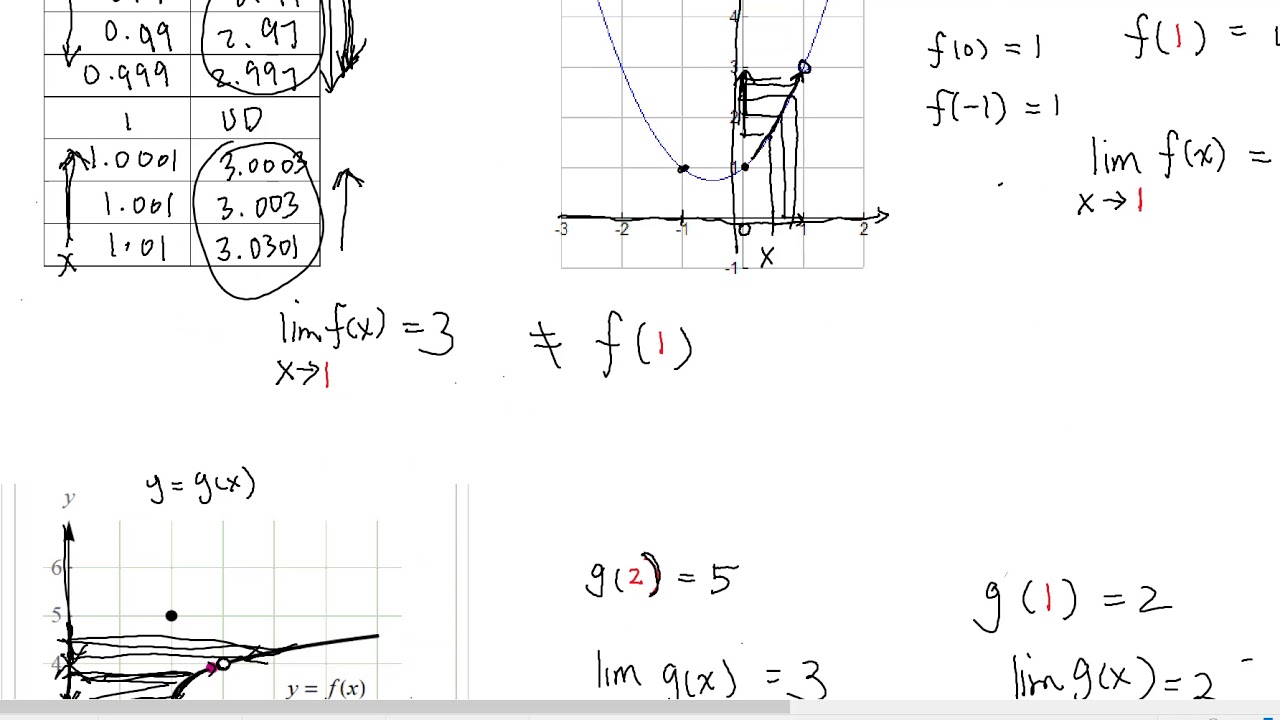
- Limit and continuity
- Differentiability
Solutions
- Exercise 2.2 | View online | Download PDF
- Exercise 2.4 | View online | Download PDF
- Exercise 2.6 | View online | Download PDF
2.2 Definition Of Derivativeap Calculus Algebra
Notes by Prof M. Tanveer
- Exercise 2.2 | View Online | Download PDF
2.2 Definition Of Derivativeap Calculus 14th Edition
- Exercise 2.5 | View Online | Download PDF